THE EQUATION OF TIME
Claudius Ptolemy, a Greek astronomer working in Alexandria around 150 CE, was an intellectual of the foremost ability. He wrote the greatest work of classical astronomy - the so-called Almagest.
This was the leading astronomical text until Copernicus some 1400 years later.
…mortal as I am, I know that I am born for a day. But when I follow the serried multitude of the Stars
in their circular course, my feet no longer touch the Earth… Ptolemy, Almagest
in their circular course, my feet no longer touch the Earth… Ptolemy, Almagest
He also wrote the defining work of Geography and another on Astrology.
Ptolemy - along with Euclid (Geometry), Galen (Medicine), Aristotle (Natural Philosophy & Cosmology) - defined what we now call science until the Enlightenment in the late 16th C and early 17th Century

The crowned Ptolemy being guided by the muse Astronomy, from Margarita Philosophica by Gregor Reisch, 1508. Throughout mediaeval times, Claudius Ptolemy was confused as one of the Ptolemies who ruled Egypt after the conquest of Alexander. Hence the crown. But also, the title ‘King Ptolemy’ is generally viewed as a mark of respect for Ptolemy's elevated standing in science.

Ptolemy, crowned again, with the globe - representing his work on Geography - with an earlier Greek astronomer Hipparchos, with the celestial globe. From Raphael's 1510 fresco 'The School of Athens.
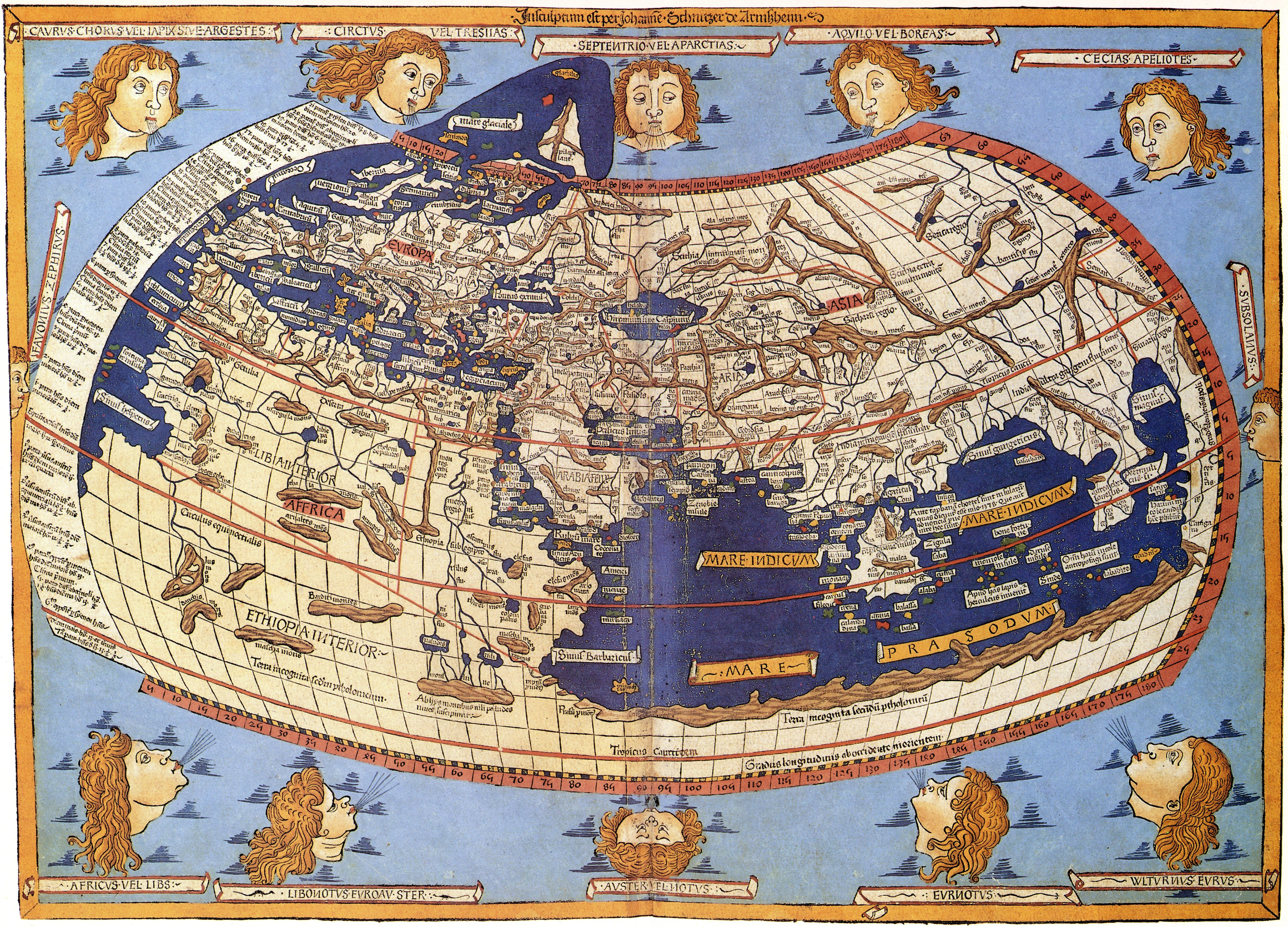
Ptolemy's - Geographia - printed 1482 British Library.
Click on images to enlarge & view captions
In his book the Almagest, without any means to directly measure the length of the day, he worked out that solar days were not of equal length. This was a feat of extraordinary intellectual vigour.
On the Inequality of Solar Days
adapted from Almagest III 9, translated by G.J. Toomer
…. it seems appropriate to add a brief discussion of the subject of the inequality of the solar day.
A grasp of this topic is a necessary prerequisite, since the mean motions which we tabulate for each body are all arranged on the simple system of equal increments, as if all solar days were of equal length. However, it can be seen that this is not so……..for two reasons:
firstly, because of the sun’s apparent anomaly:
secondly, because equal sections of the ecliptic do not cross ….. the meridian in equal times.
Neither of these effects causes a perceptible difference between the mean and the anomalistic return for a single solar day, but the accumulated difference over a number of solar days is quite noticeable……
Neglect (of these effects) would perhaps produce no perceptible error in the computation of phenomena associated with the Sun or Planets: but, in the case of the Moon, since its speed is so great, the resulting error could no longer be overlooked…
Both of these (effects) produce a maximum additive or subtractive effect, which is composed of…..
…. about 3 2/3° due to the effect of the Solar Anomaly...
…. about 4 2/3° due to the variation in the time of Meridian crossing…
In the quotation, the first reason - the ‘sun’s apparent anomaly’ - refers to the Greek's understanding of the Sun's movement in the Ecliptic, which was based on the epicyclic theory rather than Kepler's elliptical theory. This - in modern terms - describes the Eccentricity Effect. The second reason exactly describes the Obliquity Effect. Computed in modern terms, the maximum effect of the Solar Anomaly is 3.68° and that of the Meridian crossing is 4.91°
“If the call the interval between in true solar days between times t1 & t2 = Δt, and the interval between mean solar days ΔT, then Ptolemy's rule, expressed algebraically, is ΔT = Δt + E where E corresponds, in a certain sense , to the modern 'equation of time' and E = {α(t2) - α(t1)} - {λ(t2) - λ(t1)} where α = Right Ascension of the Sun about the Equator & λ is mean longitude of the Sun about the ecliptic. This rule is in fact an approximation, since one should take the motion in mean longitude, not over the interval (t2-t1) = Δt, but over the interval in mean solar days (which is in practice impossible). Since however the difference between Δt & ΔT never exceeds 33 minutes, during which the Sun moves less than 2’, the error is utterly negligible”. Quoted & adapted from Almagest III 9, translated by G.J. Toomer.
The graph of Ptolemy's Equation was derived from Robert Harry van Gent - Almagest Calculator
For those wishing to follow the extraordinary storey of the Equation of Time and Ancient Astronomy in general,, see
http://www.staff.science.uu.nl/~gent0113/astro/almagestephemeris.htm to expand your knowledge of Ptolemy calculations and see their complexities.
https://people.sc.fsu.edu/~dduke/models for many excellent animations of what the Greeks (and later astronomers) managed to achieve.
"History & Practice of Ancient Astronomy - by James Evans - Oxford University Press 1998.
"Ptolemy's Almagest" translated by G.J Toomer - Princeton Press 1998.
DIGRESSION
Ptolemy's two astronomical works, the Almagest and the accompanying Handy Tables, were the bread and butter for all astronomers for 1400 years. But they were very difficult to understand.
“If the Lord Almighty had consulted me before embarking on creation thus, I should have recommended something simpler”
King Alfonso the Wise, of Castille - 1260 AD - on discussing the Almagest
More importantly, in order to match observation with theory, Ptolemy introduced the so-called Equant Point. This was an imaginary point in the celestial sphere - now recognised to be more or less equivalent to one of the foci of the elliptical path of the earth around the sun. An imaginary point was deeply offensive to the Aristotelian philosophy of the Cosmos. Much of the effort in Islamic and Mediavel astronomy (up to and including Copernicus) was spend trying to work a geometrical system that avoided the necessity of the Equant Point
It was not until 1496, that the printed simplification of the Almagest became available was the work more ready understood.
1496 - Epytoma Ioannis de Monte Regio (Regimontanus) - Almagestum Ptolomei. Regimontanus produced a synthesis of the Almagest - which, for the first time, made the Almagest accessible to everyone.
It was not until Kepler nailed down the elliptical nature of the Earth's orbit, later proved by Newton's Laws of Gravity that Ptolemy's work - though altered somewhat by astronomers trying to get rid of the Equant point - was finally abandoned.