THE PATENTS

1867 US Patent by L.Miflin for Shaped Style Dial
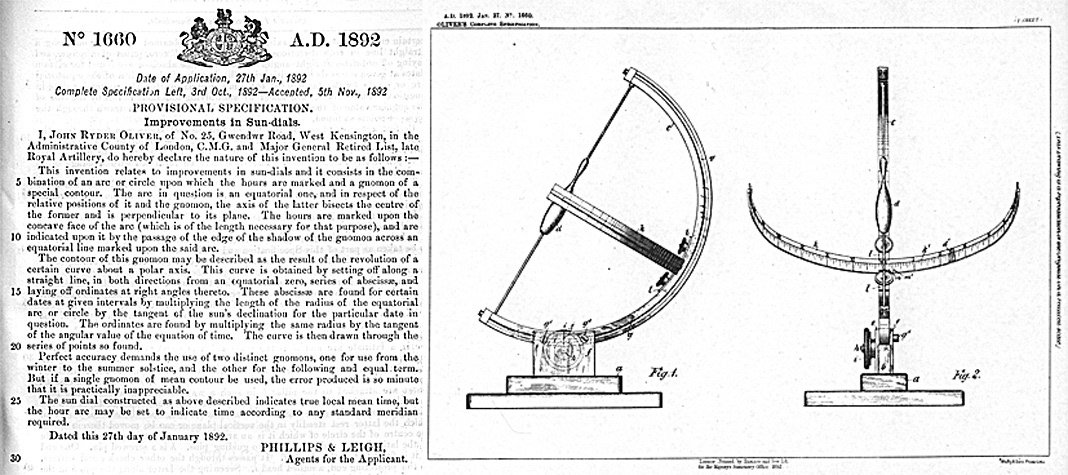
1892 UK Patent by Major John Ryder Oliver for Shaped Style Dial
Click on images to enlarge & view captions
PIERCED STYLE DIALS
In this class, the each half of the analemma is encoded in either side of the style.

Ken Clark - Schmoyer Dial 2018

3-D printed Schmoyer dial by Bill Gottesman, www.precisionsundials.com (
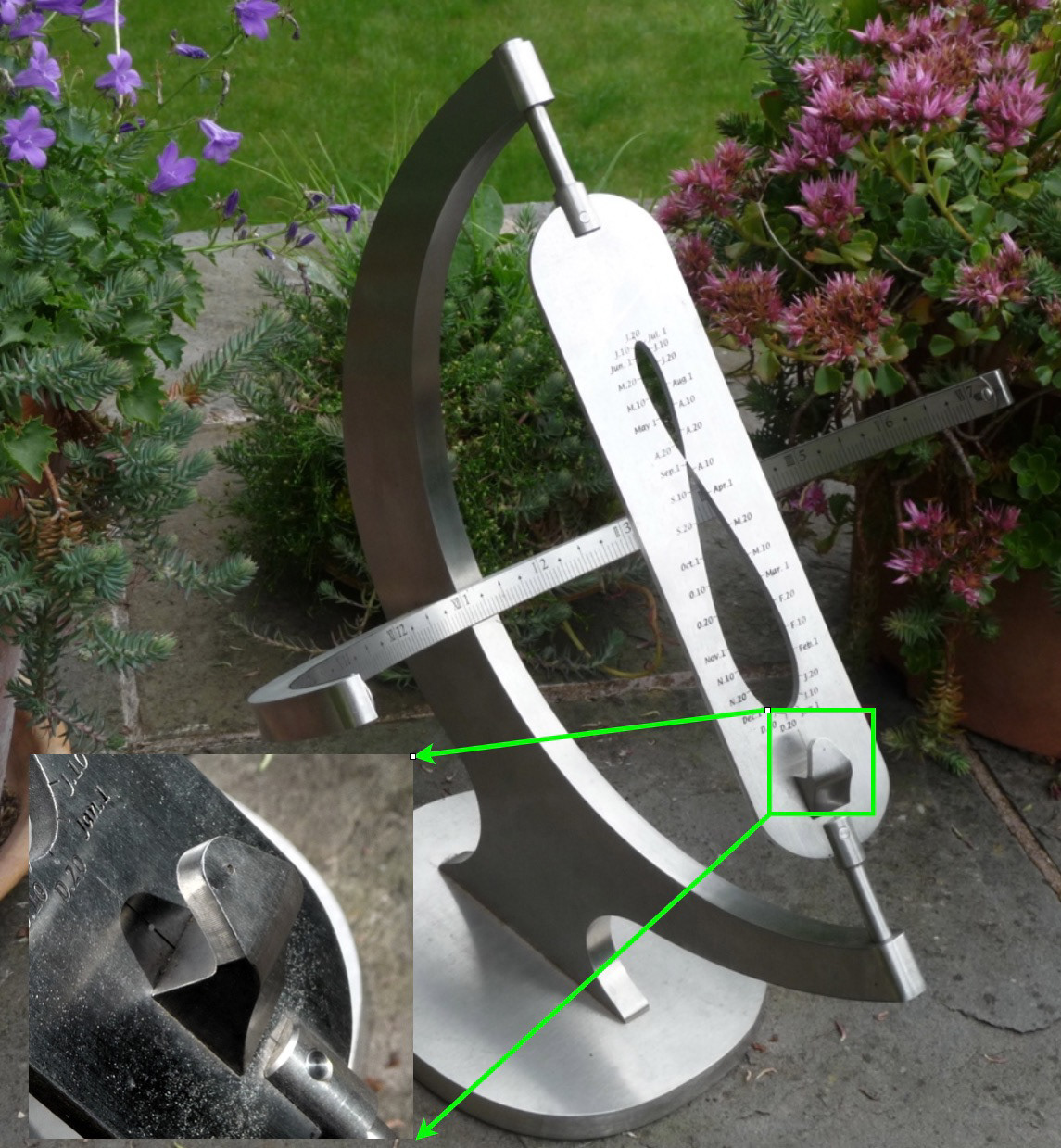
Tony Moss 'Henry Moore' dial - ca 2010 - note the clever arrangement to ensure that the plane of the analemma is perpendicular to the sun's rays

Pete Swanstrom - www.swanstrom.net/sundial/ - Ann Morrison Park, Boise, Idaho - 1998

Pete Swanstrom - www.swanstrom.net/sundial/ - New Millennium Dial - 2018
Click on images to enlarge & view captions
SHAPED STYLE DIALSIn all cases, in this class, the shape of the analemma (or half of it) is encoded in the style. If the style is symmetrical, it must encode half the analemma. In which case, two interchangeable styles must be provided) - with exceptions (see below)
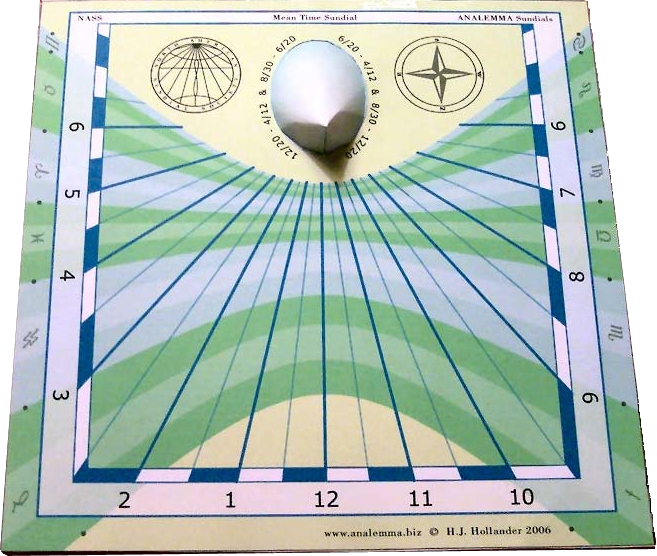
Mean Time Sundials with polar cone and vertical cone gnomons - Hendrix Hollander - www.analemma.nl - ref NASS Compendium v13(3) Sept 2006 - 2 articles : Mean Time Sundial With A Cone Gnomon & Bi-Gnomon Sundials
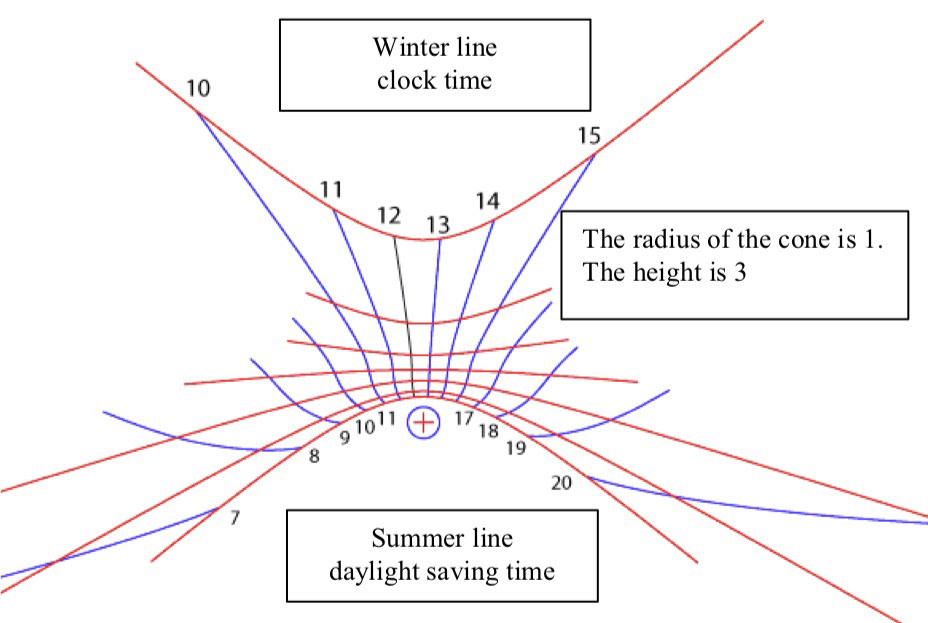
Mean Time Sundials with polar cone and vertical cone gnomons - Hendrix Hollander - www.analemma.nl - ref NASS Compendium v13(3) Sept 2006 - 2 articles : Mean Time Sundial With A Cone Gnomon & Bi-Gnomon Sundials
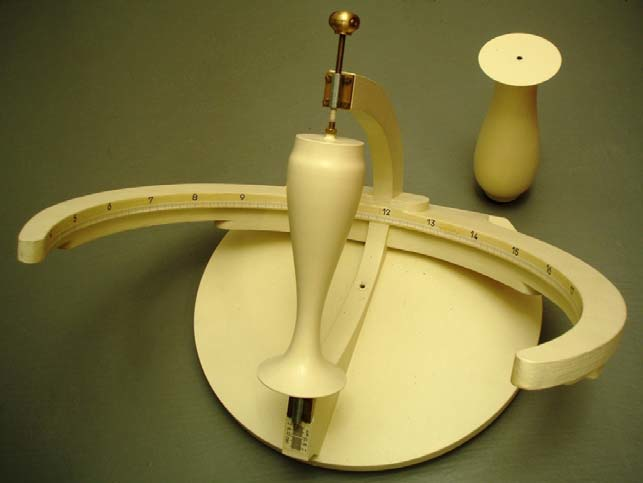
Rolf Wieland : There are two shaped styles : each representing half the analemma : Ref NASS Compendium v18(2) June 2011

Martin Bernhard' s dial at the Stuttgart Planetarium
Click on images to enlarge & view captions
THE INTEGRATED DOUBLE GNOMON SUNDIAL OF WERNER RIEGLER
This unique idea has been realised in 2003 by Werner Riegler in Joannes Kepler's city of Linz.

The solid inner and transparent outer parts of the Style

the Dial in section

Detail of the lower half of the dial

How the dial is read in the sun-ascending & sun-descending halves of the year

Werner Riegler at the installation
Click on images to enlarge & view captions
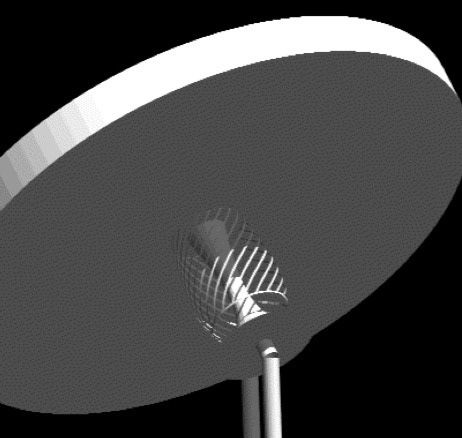
As modelled

In reality
The dial also contains a calendar. When the time is being read on one side of the dial, one of the two possible dates can be read from the shadow of the dial rim on the other side of the dial.. Click on images to enlarge & view captions

the top half of the style

the bottom half

the two previous images merged to show the analemma
Click on images to enlarge & view captions
For more detail, read below
For more information, see
ERRORS USING A SINGLE SYMMETRICAL GNOMON THROUGHOUT THE YEAR
Werner Riegler has analysed this situation in the following paper. In summary, a symmetrical gnomon can be made that will work with errors not exceeding 1.7 minutes of time. However such a gnomon will not work for some 10 days around the winter solstice and for some 2 days around the summer solstice. During these periods, the correction for one day shadows the correction of the next day. Thus, such a gnomon can only exist in the declination range -23.30° to 23.42°